Repère Orthonormé et Distance
I. Repére Orthonormé
A. Définition.
Soit (O ;I ;J) un repère du plan.
On dit que le repère (O ; I ;J) est un repère orthonormé(ou orthogonal) si les deux
droites sont perpendiculaires.
L’unité est la même sur chacun des deux axes ; OI=OJ ; i=OI et j =OJ
B. Représentation.

OI=OJ et (OI)⊥(OJ)
Repère orthonormé
II. Distance de deux points
A. Activités.
1- (O ;I ;J) est un repère orthonormé d’unité(1cm) placer les points A(2 ;4) ;B(6 ;2) et C(2 ;2).
En considérant le triangle ABC et en appliquant le théorème de Pythagore, calculer AB et trouver la valeur exacte de AB.
2- Dans un repère (O ;i ;j) orthonormé placer A($x_{A}$;$y_{A}$) B($x_{B}$ ;$y_{B}$) ; C($x_{C}$ ;$y_{C}$).
Exprimer $BC^{2}$ en fonction de $x_{A}$ et $x_{B}$.
Exprimer $AC^{2}$ en fonction de $y_{A}$ et $y_{B}$. Calculer $AB^{2}$ .
En déduire AB en fonction de $x_{A}$ et $x_{B}$ ; $y_{A}$ et $y_{B}$.


$AB^{2}$ = $AC^{2}$ + $BC^{2}$
AC = | 4 - 2 | = 2
BC = | 6 - 2 | = 4
$AB^{2}$ = 22 + 42 = 4 + 16 = 20
AB = $\sqrt{20} = 2\sqrt{5}$
$BC^{2} = (x_{B} - x_{A})^{2}$
$AC^{2} = (y_{A} - y_{B})^{2}$
Calculons $AB^{2}$
$AB^{2} = BC^{2} + AC^{2} = (x_{B} - x_{A})^{2} + (y_{A} - y_{B})^{2}$
AB = $\sqrt{(x_{B} − x_{A})^{2} + (y_{A} − y_{B})^{2}}$
B. Définition.
La distance des points $A(x_{A} ;y_{A}) et B(x_{B} ;y_{B})$ du plan muni d'unrepère orthonormé est : AB = $\sqrt{(x_{B} − x_{A})^{2} + (y_{A} − y_{B})^{2}}$
Exercice d’application
Le plan est muni d’un repère orthonormé (O ;i ;j).
1- Placer les points A(-1 ;3) ;B(-2 ;1) ;C(3 ;1).
2- Démontrer que le triangle ABC est rectangle et préciser en quel point.
III. Orthogonalités de deux vecteurs
A. Représentation.
Soit $\overrightarrow{u}$ et $\overrightarrow{v}$ deux vecteurs non nuls tels que : $\overrightarrow{u}$ = $\overrightarrow{AB}$ et $\overrightarrow{v}$ = $\overrightarrow{AC}$ et (AB) $\perp (AC)$
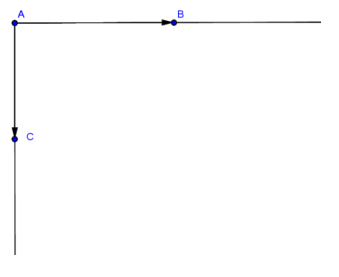
B. Définition.
$\overrightarrow{u}$ et $\overrightarrow{v}$ sont orthogonaux signifie que les droites (AB ) et ( AC)sont perpendiculaires.
C. Caractérisation de l'orthogonalités de deux vecteurs non nuls.
Dans un repère orthonormé : $\overrightarrow{u}$ $ \left(\begin{array}{clcr}x \\ y \end{array}\right) $ et $\overrightarrow{v}$ $ \left(\begin{array}{clcr}x' \\ y' \end{array}\right) $ étant deux vecteurs non nuls:
- Si $\overrightarrow{u}$ et $\overrightarrow{v}$ sont orthogonaux alors ; $xx’+yy’=0$ et réciproquement.
- Si $xx’+yy’=0$ alors $\overrightarrow{u}$ et $\overrightarrow{v}$ sont orthogonaux.
Conséquence du théorème
Dans un repère orthonormé : $\overrightarrow{u}$ $ \left(\begin{array}{clcr}x \\ y \end{array}\right) $ et $\overrightarrow{v}$ $ \left(\begin{array}{clcr}x' \\ y' \end{array}\right) $ étant deux vecteurs non nuls.
Si $xx’+yy’ \ne$ 0, alors $\overrightarrow{u}$ et $\overrightarrow{v}$ ne sont pas orthogonaux.